All Real Numbers Domain Example

It is the set of all values for which a function is mathematically defined. The domain of the function f x 1 x is all real numbers except zero since at x 0 the function is undefined.

Real Numbers Videos Part 1 Digital Learning Real Numbers Digital Learning Number Videos

For example the domain of f xx² is all real numbers and the domain of g x1x is all real numbers except for x0.

All real numbers domain example. LIM2B1 EK LIM2B2 EK Transcript. If an inequality would be true for all possible values the answer is all real numbers. The range is also all real numbers except zero.

In other words given the composite fgx the domain will exclude all values where. We can also define special functions whose domains are more limited. Real numbers are simply the combination of rational and irrational numbers in the number system.

No matter how big or how small. The domain of the f gx consists of all x-values that are in the domain of both f and g. It is the distance from 0 on the number line.

So the domain of the function is x 0. All real numbers 100. Hence the domain is all real x except x2 and x3.

Therefore this statement can be. It is quite common for the domain to be the set of all real numbers since many mathematical functions can accept any input. The domain and range are all real numbers because at some point the x and y values will be every real number.

The Quotient of Two Functions. When using set notation inequality symbols such as are used to describe the domain and range. The domain of f x x 2 - 6 is also because f x is defined for all real numbers x.

Confirming continuity over an interval. You can check that the vertex is indeed at 1 4. The domain of.

Solving Inequalities with No Solution or All Real Numbers. Find the domain and range of the following function. Some functions however are not defined for all the real numbers and thus are evaluated over a restricted domain.

For this one - the graph simply does not exist in the negative domain. For example the function. The domain of a function is the set of all possible inputs for the function.

The domain of f x is. For example in the toolkit functions we introduced the absolute value function With a domain of all real numbers and a range of values greater than or equal to 0 absolute value can be defined as the magnitude or modulus of a real number value regardless of sign. Find the domain of f x x 3 x 2.

We can see that this function has horizontal values that start at and extend indefinitely to the right. Suppose we have two functions. Since a quadratic function has two mirror image halves the line of reflection has to be in the middle of two points with the same y value.

The set of all real numbers x such that x is greater than 2 and less than or equal to 3 As stated above we can use set-builder notation to express the domain of a function. The domain is all real numbers and the range is all real numbers fx such that fx 4. In a graph around these points the value of y will go to infinity.

The domain is all real numbers because there is no restriction for the value of x or the input. What every function actually does is simple. Sal is asked which of the following two functions is continuous on all real numbers.

For every member from the domain the function chooses exactly one member from the codomain. In this example f has domain x x 0 and g has domain all real numbers therefore f gx has domain x x 0 because these values of x are in the domain of both f and g. Y y 0 R indicates range.

In general the common functions are continuous on all the numbers in their domain. The domain of a composite must exclude all values that make the inside function undefined and all values that make the composite function undefined. For example the domain of f x is because we cannot take the square root of a negative number.

All real numbers Range. However we see that the function has an asymptote at that is the function never takes that valueTherefore the domain of the function is all real numbers of x where and. Also what does domain of all real numbers mean.

Has domain that consists of all real numbers greater than or equal to zero because the square root of a negative number is not a. The correct answer is Domain. Y x 12 Here when x.

Division by zero is not allowed. Answer 1 of 5. The domain of a function is the set of all values for which the function is defined.

The function is not defined for since this value would produce a division by 0. In general all the arithmetic operations can be performed on these numbers and they can be represented in the number line also. The line- and function- to the left has a domain and range of all real numbers because as the arrows indicate the graph goes on forever both negatively and positively.

For most functions in algebra the domain is the set of all real numbers. The set of all possible input values commonly the x variable which produce a valid output from a particular function. This equation is in a factored form.

At the same time the imaginary numbers are the un-real numbers which cannot be expressed in the number line and is commonly used to represent a complex number. Putting it all together this statement can be read as the domain is the set of all x such that x is an element of all real numbers The range of fx x 2 in set notation is. Because the equation is in a factored form the.

Find the domain and range for the function. It is very important to understand. For example lets choose as a domain the set of all your Fb friends.

But there are two cases where this is not always true fractions with a variable in the denominator and radicals with an even index. If the inequality states something untrue there is no solution. It can be literally every set.

Therefore the domain of the function is all real numbers with the exception of -5.

Functions 6 Modulus Functions And Solving Modulus Equations Worksheet Mathlessons Math Elementarymath Mathcen Teaching Math Math Classroom Equations

Example 4 Graph A Translated Square Root Function Graph Y 2 X 3 2 Then State The Domain And Range Solution Step Graphing Quadratics Function Of Roots

Pin On Algebra

Linear Quadratic Exponential Tables Worksheet Graphing Linear Equations Graphing Quadratics Quadratics

Identifying Polynomial Functions By Degrees Polynomial Functions Polynomials Real Numbers

Identifying Polynomial Functions By Degrees Polynomial Functions Polynomials Real Numbers

Domain And Range From Graph Khan Academy Interpreting Motion Graphs Solving Quadratic Equations Algebra Worksheets

Pin By The Radiance Room On Math Positive Numbers Real Numbers Positivity

Pin On Math Explorations

Range And Domain Rational Function Informative Domain

Exploring Inverses Of Functions With Desmos Inverse Functions Quadratics Functions Algebra

Pin By Math Teacher On Algebra Quadratics Math School High School Math Classroom

Graphing Quadratic Equations Quadratics Quadratic Functions Quadratic Equation

Domain And Range Anchor Chart Poster Anchor Charts Chart Teaching Math

Function Operation Adding And Subtracting Adding And Subtracting Subtraction Real Numbers

Pin On Algebra

Practice Domain And Range Of Absolute Value Functions With Ixl 20 Free Questions Per Day Algebra 1 Absolute Value Solving Quadratic Equations

Pin By Nur Jamaludin On Functions Math Functions Math Mathematics Linear Function

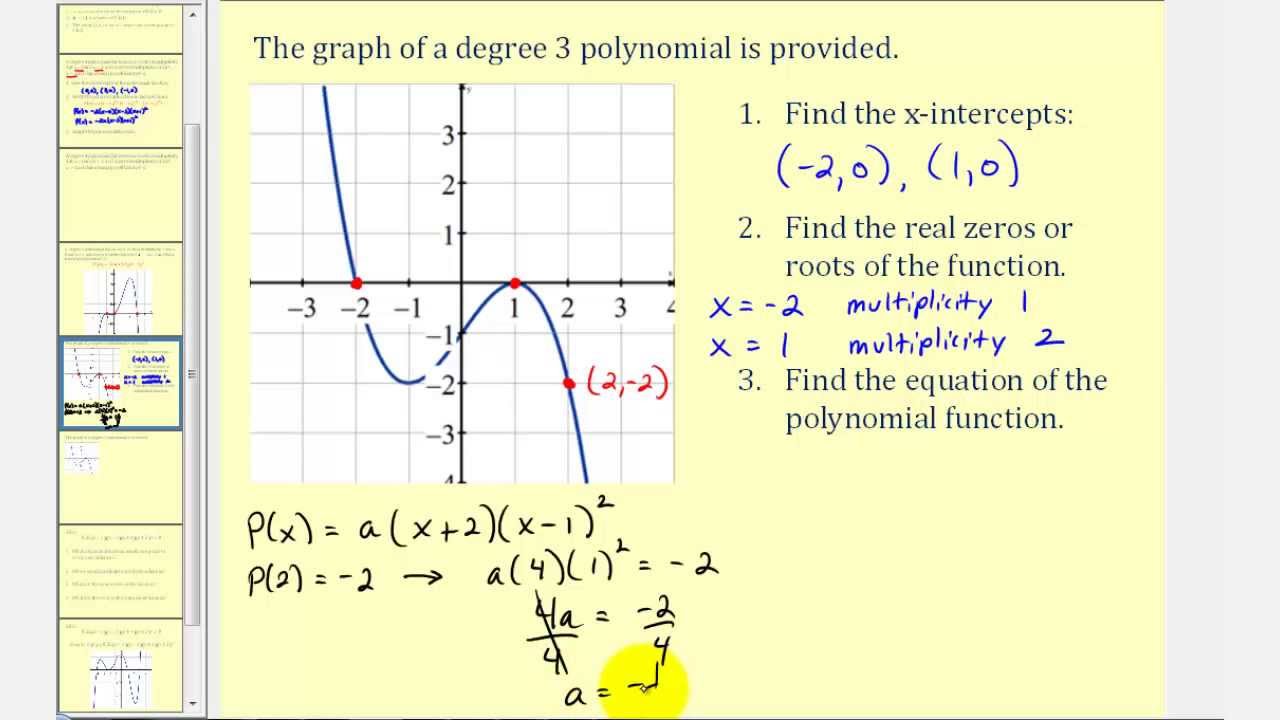
Real Zeros Factors And Graphs Of Polynomial Functions Polynomials Graphing Quadratics Graphing Linear Equations Activities